Buses are powered by generators, and charges are used to take energy from them. The buses are nodes in the power system network, allowing for the definition of a voltage for each bus. A bus is a node in a power grid that links one or more lines while also containing various components such as loads and generators. One of four parameters is associated with each bus or node. (1) Voltage phase angle, (2) voltage magnitude, (3) reactive power, and (4) true or active power.

In a Power System buses are classified into three categories based on the quantity to be specified: generation bus, load bus, and slack bus.

Buses receive energy from generators, and when buses receive energy from generators the buses become nodes in a power system’s network, allowing a voltage to be specified for each bus.

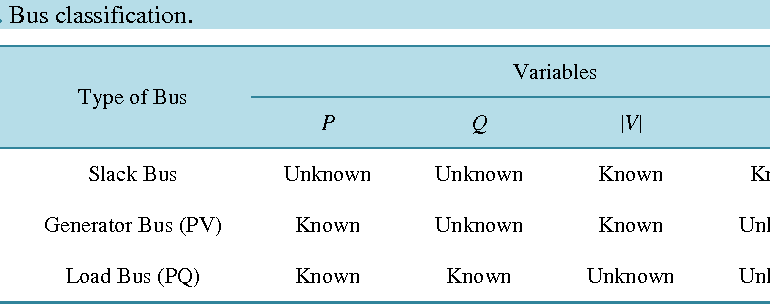
Load Bus:

PQ buses are another name for load buses. This bus is equipped with both real and reactive power. The magnitude and phase angles of the voltage are unknown. It can be found using a load flow solution and a variety of ways. The voltage on a load bus is allowed to vary within permissible limits.

Generator bus:

Buses that run on generators are often known as voltage-controlled buses or PV buses. It is used to connect the generator to the generating transformer. In this form of bus, the natural power generation and voltage magnitude are defined. The load flow equation must be solved in order to determine the voltage and reactive power level of the generator bus.

Slack Bus:

A slack system can be used as a swing bus. The phase and voltage of a slack bus are defined. A slack bus is the initial bus in a power system. It’s because no load flow analysis can be done without a slack bus. The slack bus can also be used as a reference bus for load flow solutions. The slack bus is usually served by a single generator bus.

Load flow analysis

The mathematical model for the load flow analysis is differential equations. It also serves as the foundation for dynamic analysis, which aids in the comprehension of power systems. As a result, understanding approaches that make power systems operational and efficient necessitates a thorough comprehension of load flow theories and algorithms.